Read: Solve Compound Inequalities—OR
Learning Objectives
- Solve compound inequalities in the form of or and express the solution graphically and with an interval
Solve compound inequalities in the form of or
As we saw in the last section, the solution of a compound inequality that consists of two inequalities joined with the word or is the union of the solutions of each inequality. Unions allow us to create a new set from two that may or may not have elements in common. TIP: When finding the solutions to compound inequalities with the word OR, you are including all answers for each inequality in your new solution. This is easiest to see when both are graphed on a number line. It is possible to have the entire number line shaded, just a portion shaded, or both ends shaded with a non-shaded region in the middle. In this section you will see that some inequalities need to be simplified before their solution can be written or graphed. In the following example, you will see an example of how to solve a one-step inequality in the OR form. Note how each inequality is treated independently until the end where the solution is described in terms of both inequalities. You will use the same properties to solve compound inequalities that you used to solve regular inequalities.Example
Solve for x. [latex]3x–1<8[/latex] or [latex]x–5>0[/latex]Answer: Solve each inequality by isolating the variable.
[latex] \displaystyle \begin{array}{r}x-5>0\,\,\,\,\,\,\,\,\text{OR}\,\,\,\,\,\,\,\,\,\,3x-1<8\\\underline{\,\,\,+5\,\,+5}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\underline{\,\,\,\,\,\,+1\,+1}\\x\,\,\,\,\,\,>5\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\underline{3x}\,\,\,<\underline{9}\\{3}\,\,\,\,\,\,\,\,\,\,\,\,\,{3}\\x<3\,\,\,\\x>5\,\,\,\,\text{or}\,\,\,\,x<3\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\end{array}[/latex]
Write both inequality solutions as a compound using or, using interval notation.Answer
Inequality: [latex] \displaystyle x>5\,\,\,\,\text{or}\,\,\,\,x<3[/latex] Interval: [latex]\left(-\infty, 3\right)\cup\left(5,\infty\right)[/latex] The solution to this compound inequality can also be shown graphically. Sometimes it helps to draw the graph first before writing the solution using interval notation.
Example
Solve for y. [latex]2y+7\lt13\text{ or }−3y–2\lt10[/latex]Answer: Solve each inequality separately.
[latex]\begin{equation}\begin{aligned}2y+7<13\quad\quad\text{OR}\quad\quad-3y-2\lt 10\\\underline{\quad-7\quad-7}\quad\quad\quad\quad\quad\quad\quad\underline{\quad+2\quad+2}\\\underline{2y}<\underline{6}\quad\quad\quad\quad\quad\quad\quad\quad\underline{-3y}\lt\underline{12}\\2\quad\quad2\quad\quad\quad\quad\quad\quad\quad\quad-3\quad-3\\y<3\quad\quad\quad\quad\quad\quad\quad\quad\quad{y}\gt -4\end{aligned}\end{equation}[/latex]
The inequality sign is reversed with division by a negative number. Since y could be less than [latex]3[/latex] or greater than [latex]−4[/latex], y could be any number. Graphing the inequality helps with this interpretation.Answer
Inequality: [latex]y<3\text{ or }y> -4[/latex] Interval: [latex]\left(-\infty,\infty\right)[/latex] Graph: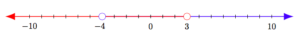 Even though the graph shows empty dots at [latex]y=3[/latex] and [latex]y=-4[/latex], they are included in the solution.
Even though the graph shows empty dots at [latex]y=3[/latex] and [latex]y=-4[/latex], they are included in the solution.
Example
Solve for [latex]z[/latex]. [latex-display]5z–3\gt−18[/latex] or [latex]−2z–1\gt15[/latex-display]Answer: Solve each inequality separately. Combine the solutions.
[latex] \displaystyle \begin{array}{l}5z-3>-18\,\,\,\,\,\,\,\,\,\text{OR}\,\,\,\,\,\,\,-2z-1>15\\\underline{\,\,\,\,\,\,+3\,\,\,\,\,\,\,+3}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\underline{\,\,\,\,\,\,\,\,\,+1\,\,\,\,+1}\\\Large\frac{5z}{5}\,\,\,\,\,\,\,\,>\,\frac{-15}{5}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\frac{-2z}{-2}\,\,\,\,\,\,<\,\,\frac{16}{-2}\\\,\,\,\,\,\,\,\,\,\,z>-3\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,z<-8\\\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,z>-3\,\,\,\,\text{or}\,\,\,\,z<-8\end{array}[/latex]
Answer
Inequality: [latex] \displaystyle z>-3\,\,\,\,\text{or}\,\,\,\,z<-8[/latex] Interval: [latex]\left(-\infty,-8\right)\cup\left(-3,\infty\right)[/latex] Note how we write the intervals with the one containing the most negative solutions first, then move to the right on the number line. [latex]z<-8[/latex] has solutions that continue all the way to the left on the number line, whereas [latex]x>-3[/latex] has solutions that continue all the way to the right. In this way we write solutions with intervals from left to right. Graph:
Licenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- Ex: Solve a Compound Inequality Involving OR (Union). Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Unit 10: Solving Equations and Inequalities, from Developmental Math: An Open Program. Provided by: Monterey Institute of Technology and Education Located at: https://www.nroc.org/. License: CC BY: Attribution.
