We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > Intermediate Algebra

Read: Solution Sets of Inequalities

Learning Objectives

  • Identify whether an ordered pair is in the solution set of a linear inequality
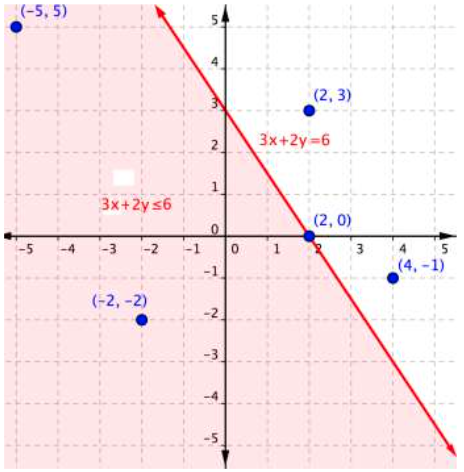The graph below shows the region of values that makes the inequality [latex]3x+2y\leq6[/latex] true (shaded red), the boundary line [latex]3x+2y=6[/latex], as well as a handful of ordered pairs. The boundary line is solid because points on the boundary line [latex]3x+2y=6[/latex] will make the inequality [latex]3x+2y\leq6[/latex] true. A solid downward-sloping line running. The region below the line is shaded and is labeled 3x+2y is less than or equal to 6. The region above the line is unshaded and is labeled 3x+2y=6. The points (-5,5) and (-2,-2) are in the shaded region. The points (2,3) and (4,-1) are in the unshaded region. The point (2,0) is on the line. You can substitute the x- and y-values in each of the [latex](x,y)[/latex] ordered pairs into the inequality to find solutions. Sometimes making a table of values makes sense for more complicated inequalities.
Ordered Pair Makes the inequality [latex-display]3x+2y\leq6[/latex-display] a true statement Makes the inequality [latex-display]3x+2y\leq6[/latex-display] a false statement
[latex](−5, 5)[/latex] [latex]\begin{array}{r}3\left(−5\right)+2\left(5\right)\leq6\\−15+10\leq6\\−5\leq6\end{array}[/latex]
[latex](−2,−2)[/latex] [latex]\begin{array}{r}3\left(−2\right)+2\left(–2\right)\leq6\\−6+\left(−4\right)\leq6\\–10\leq6\end{array}[/latex]
[latex](2,3)[/latex] [latex]\begin{array}{r}3\left(2\right)+2\left(3\right)\leq6\\6+6\leq6\\12\leq6\end{array}[/latex]
[latex](2,0)[/latex] [latex]\begin{array}{r}3\left(2\right)+2\left(0\right)\leq6\\6+0\leq6\\6\leq6\end{array}[/latex]
[latex](4,−1)[/latex] [latex]\begin{array}{r}3\left(4\right)+2\left(−1\right)\leq6\\12+\left(−2\right)\leq6\\10\leq6\end{array}[/latex]
If substituting [latex](x,y)[/latex] into the inequality yields a true statement, then the ordered pair is a solution to the inequality, and the point will be plotted within the shaded region or the point will be part of a solid boundary line. A false statement means that the ordered pair is not a solution, and the point will graph outside the shaded region, or the point will be part of a dotted boundary line.    

Example

Use the graph to determine which ordered pairs plotted below are solutions of the inequality [latex]x–y<3[/latex].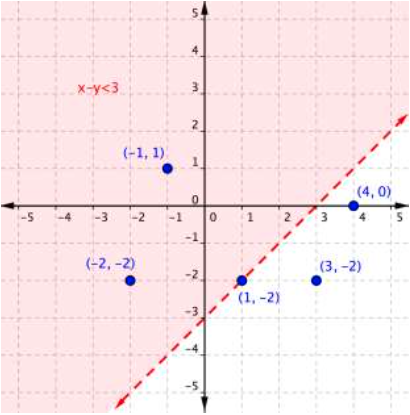 Upward-sloping dotted line. The region above the line is shaded and labeled x-y<3. The points (4,0) and (3,-2) are in the unshaded region. The point (1,-2) is on the dotted line. The points (-1,1) and (-2,-2) are in the shaded region.

Answer: Solutions will be located in the shaded region. Since this is a “less than” problem, ordered pairs on the boundary line are not included in the solution set. These values are located in the shaded region, so are solutions. (When substituted into the inequality [latex]x–y<3[/latex], they produce true statements.)

[latex](−1,1)[/latex]

[latex](−2,−2)[/latex]

These values are not located in the shaded region, so are not solutions. (When substituted into the inequality [latex]x-y<3[/latex], they produce false statements.)

[latex](1,−2)[/latex]

[latex](3,−2)[/latex]

[latex](4,0)[/latex]

Answer

[latex](−1,1)\,\,\,(−2,−2)[/latex]

The following video show an example of determining whether an ordered pair is a solution to an inequality. https://youtu.be/GQVdDRVq5_o

Example

Is [latex](2,−3)[/latex] a solution of the inequality [latex]y<−3x+1[/latex]?

Answer: If [latex](2,−3)[/latex] is a solution, then it will yield a true statement when substituted into the inequality [latex]y<−3x+1[/latex].

[latex]y<−3x+1[/latex]

Substitute [latex]x=2[/latex] and [latex]y=−3[/latex] into inequality.

[latex]−3<−3\left(2\right)+1[/latex]

Evaluate.

[latex]\begin{array}{l}−3<−6+1\\−3<−5\end{array}[/latex]

This statement is not true, so the ordered pair [latex](2,−3)[/latex] is not a solution.

Answer

[latex](2,−3)[/latex] is not a solution.

The following video shows another example of determining whether an ordered pair is a solution to an inequality. https://youtu.be/-x-zt_yM0RM

Licenses & Attributions

CC licensed content, Shared previously

  • Use a Graph Determine Ordered Pair Solutions of a Linear Inequalty in Two Variable. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
  • Ex: Determine if Ordered Pairs Satisfy a Linear Inequality. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
  • Unit 13: Graphing, from Developmental Math: An Open Program. Provided by: Monterey Institute of Technology Located at: https://www.nroc.org/. License: CC BY: Attribution.