Solutions
Solutions to Try Its
1. a. yes; b. yes. (Note: If two players had been tied for, say, 4th place, then the name would not have been a function of rank.) 2. [latex]g\left(5\right)=1[/latex] 3. [latex]m=8[/latex] 4. [latex]y=f\left(x\right)=\frac{\sqrt[3]{x}}{2}[/latex] 5. [latex]g\left(1\right)=8[/latex] 6. [latex]x=0[/latex] or [latex]x=2[/latex] 7. a. yes, because each bank account has a single balance at any given time; b. no, because several bank account numbers may have the same balance; c. no, because the same output may correspond to more than one input. 8. yesSolutions to Odd-Numbered Exercises
1. A relation is a set of ordered pairs. A function is a special kind of relation in which no two ordered pairs have the same first coordinate. 3. When a vertical line intersects the graph of a relation more than once, that indicates that for that input there is more than one output. At any particular input value, there can be only one output if the relation is to be a function. 5. When a horizontal line intersects the graph of a function more than once, that indicates that for that output there is more than one input. A function is one-to-one if each output corresponds to only one input. 7. function 9. function 11. function 13. function 15. function 17. function 19. function 21. function 23. function 25. not a function 27. [latex]f\left(-3\right)=-11\\ f\left(2\right)=-1\\ f\left(-a\right)=-2a - 5\\-f\left(a\right)=-2a+5\\f\left(a+h\right)=2a+2h - 5\\[/latex] 29. [latex]f\left(-3\right)=\sqrt{5}+5\\ f\left(2\right)=5\\ f\left(-a\right)=\sqrt{2+a}+5\\ -f\left(a\right)=-\sqrt{2-a}-5\\f\left(a+h\right)=\\sqrt{2-a-h}+5\\[/latex] 31. [latex]f\left(-3\right)=2\\f\left(2\right)=1 - 3=-2\\f\left(-a\right)=|-a - 1|-|-a+1|\\ -f\left(a\right)=-|a - 1|+|a+1|\\\text{ }\text{ }f\left(a+h\right)=|a+h - 1|-|a+h+1|\\[/latex] 33. [latex]\frac{g\left(x\right)-g\left(a\right)}{x-a}=x+a+2,x\ne a[/latex] 35. a. [latex]f\left(-2\right)=14[/latex]; b. [latex]x=3[/latex] 37. a. [latex]f\left(5\right)=10[/latex]; b. [latex]x=-1\text{ }[/latex] or [latex]\text{ }x=4[/latex] 39. a. [latex]f\left(t\right)=6-\frac{2}{3}t[/latex]; b. [latex]f\left(-3\right)=8[/latex]; c. [latex]t=6[/latex] 41. not a function 43. function 45. function 47. function 49. function 51. function 53. a. [latex]f\left(0\right)=1[/latex]; b. [latex]f\left(x\right)=-3,x=-2\text{ }[/latex] or [latex]\text{ }x=2[/latex] 55. not a function so it is also not a one-to-one function 57. one-to-one function 59. function, but not one-to-one 61. function 63. function 65. not a function 67. [latex]f\left(x\right)=1,x=2[/latex] 69. [latex]f\left(-2\right)=14\\ f\left(-1\right)=11\\ f\left(0\right)=8\\f\left(1\right)=5\\ f\left(2\right)=2\\[/latex] 71. [latex]f\left(-2\right)=4\\\text{ }\\ f\left(-1\right)=4.414\\ f\left(0\right)=4.732\\ f\left(1\right)=4.5\\ f\left(2\right)=5.236\\[/latex] 73. [latex]f\left(-2\right)=\frac{1}{9}\\ f\left(-1\right)=\frac{1}{3}\\ f\left(0\right)=1\\ f\left(1\right)=3;\\f\left(2\right)=9\\[/latex] 75. 20 77. [latex]\left[0,\text{ 100}\right][/latex] 79.[latex]\left[-0.001,\text{ 0}\text{.001}\right][/latex]
79.[latex]\left[-0.001,\text{ 0}\text{.001}\right][/latex]
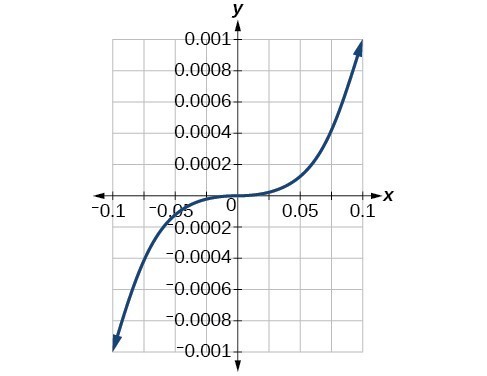 81. [latex]\left[-1,000,000,\text{ 1,000,000}\right][/latex]
81. [latex]\left[-1,000,000,\text{ 1,000,000}\right][/latex]
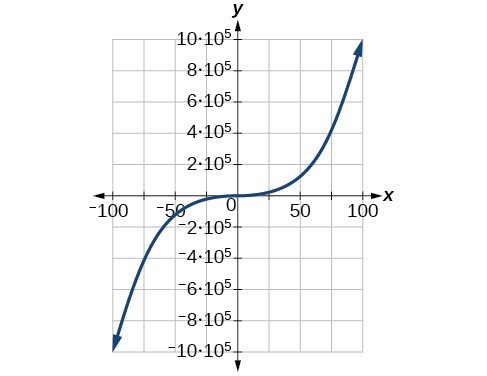 83. [latex]\left[0,\text{ 10}\right][/latex]
83. [latex]\left[0,\text{ 10}\right][/latex]
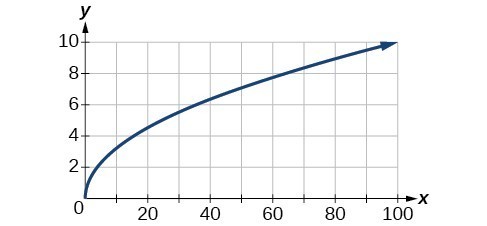 85. [latex]\left[-0.1,\text{0.1}\right][/latex]
85. [latex]\left[-0.1,\text{0.1}\right][/latex]
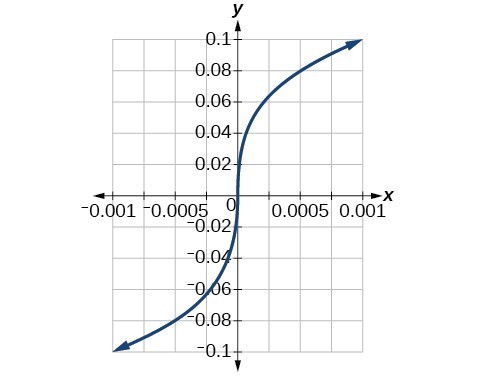 87. [latex]\left[-100,\text{ 100}\right][/latex]
87. [latex]\left[-100,\text{ 100}\right][/latex]
 89. a. [latex]g\left(5000\right)=50[/latex]; b. The number of cubic yards of dirt required for a garden of 100 square feet is 1.
91. a. The height of a rocket above ground after 1 second is 200 ft. b. the height of a rocket above ground after 2 seconds is 350 ft.
89. a. [latex]g\left(5000\right)=50[/latex]; b. The number of cubic yards of dirt required for a garden of 100 square feet is 1.
91. a. The height of a rocket above ground after 1 second is 200 ft. b. the height of a rocket above ground after 2 seconds is 350 ft.Licenses & Attributions
CC licensed content, Shared previously
- Precalculus. Provided by: OpenStax Authored by: Jay Abramson, et al.. Located at: https://openstax.org/books/precalculus/pages/1-introduction-to-functions. License: CC BY: Attribution. License terms: Download For Free at : http://cnx.org/contents/[email protected]..

