We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > Precalculus II

Solutions for Modeling with Trigonometric Equations

Solutions to Try Its

1. The amplitude is [latex]\text{ }3[/latex], and the period is [latex]\text{ }\frac{2}{3}[/latex]. 2.
x [latex]3\sin \left(3x\right)[/latex]
0 0
[latex]\frac{\pi }{6}[/latex] 3
[latex]\frac{\pi }{3}[/latex] 0
[latex]\frac{\pi }{2}[/latex] [latex]-3[/latex]
[latex]\frac{2\pi }{3}[/latex] 0
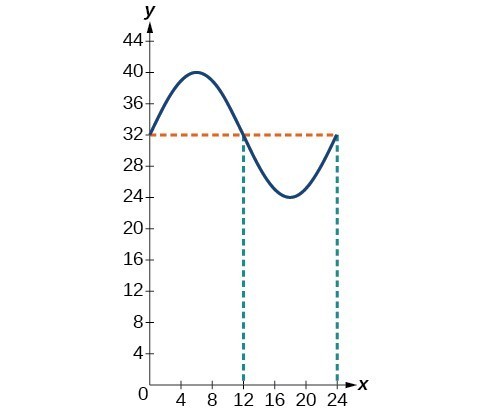
Graph of y=3sin(3x) using the five key points: intervals of equal length representing 1/4 of the period. Here, the points are at 0, pi/6, pi/3, pi/2, and 2pi/3. 3. [latex]y=8\sin \left(\frac{\pi }{12}t\right)+32[/latex] The temperature reaches freezing at noon and at midnight. Graph of the function y=8sin(pi/12 t) + 32 for temperature. The midline is at 32. The times when the temperature is at 32 are midnight and noon. 4. initial displacement =6, damping constant = -6, frequency = [latex]\frac{2}{\pi }[/latex] 5. [latex]y=10{e}^{-0.5t}\cos \left(\pi t\right)[/latex] 6. [latex]y=5\cos \left(6\pi t\right)[/latex]

Solutions to Odd-Numbered Exercises

1. Physical behavior should be periodic, or cyclical. 3. Since cumulative rainfall is always increasing, a sinusoidal function would not be ideal here. 5. [latex]y=-3\cos \left(\frac{\pi }{6}x\right)-1[/latex] 7. [latex]5\sin \left(2x\right)+2[/latex] 9. [latex]4\cos \left(\frac{x\pi }{2}\right)-3[/latex] 11. [latex]5 - 8\sin \left(\frac{x\pi }{2}\right)[/latex] 13. [latex]\tan \left(\frac{x\pi }{12}\right)[/latex] 15. Answers will vary. Sample answer: This function could model temperature changes over the course of one very hot day in Phoenix, Arizona. Graph of f(x) = -18cos(x*pi/12) - 5sin(x*pi/12) + 100 on the interval [0,24]. There is a single peak around 12. 17. 9 years from now 19. [latex]56^\circ \text{F}[/latex] 21. [latex]1.8024[/latex] hours 23. 4:30 25. From July 8 to October 23 27. From day 19 through day 40 29. Floods: July 24 through October 7. Droughts: February 4 through March 27 31. Amplitude: 11, period: [latex]\frac{1}{6}[/latex], frequency: 6 Hz 33. Amplitude: 5, period: [latex]\frac{1}{30}[/latex], frequency: 30 Hz 35. [latex]P\left(t\right)=-15\cos \left(\frac{\pi }{6}t\right)+650+\frac{55}{6}t[/latex] 37. [latex]P\left(t\right)=-40\cos \left(\frac{\pi }{6}t\right)+800{\left(1.04\right)}^{t}[/latex] 39. [latex]D\left(t\right)=7{\left(0.89\right)}^{t}\cos \left(40\pi t\right)[/latex] 41. [latex]D\left(t\right)=19{\left(0.9265\right)}^{t}\cos \left(26\pi t\right)[/latex] 43. [latex]20.1[/latex] years 45. 17.8 seconds 47. Spring 2 comes to rest first after 8.0 seconds. 49. 500 miles, at [latex]{90}^{\circ }[/latex] 51. [latex]y=6{\left(5\right)}^{x}+4\sin \left(\frac{\pi }{2}x\right)[/latex] 53. [latex]y=8{\left(\frac{1}{2}\right)}^{x}\cos \left(\frac{\pi }{2}x\right)+3[/latex]

Licenses & Attributions

CC licensed content, Specific attribution