We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > Precalculus I

Solutions 24: Graphs of Logarithmic Functions

Solutions to Try Its

1. [latex]\left(2,\infty \right)[/latex] 2. [latex]\left(5,\infty \right)[/latex] 3. The domain is [latex]\left(0,\infty \right)[/latex], the range is [latex]\left(-\infty ,\infty \right)[/latex], and the vertical asymptote is x = 0.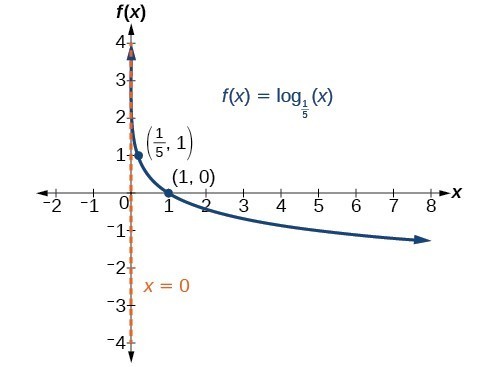 Graph of f(x)=log_(1/5)(x) with labeled points at (1/5, 1) and (1, 0). The y-axis is the asymptote. 4. The domain is [latex]\left(-4,\infty \right)[/latex], the range [latex]\left(-\infty ,\infty \right)[/latex], and the asymptote x = –4. 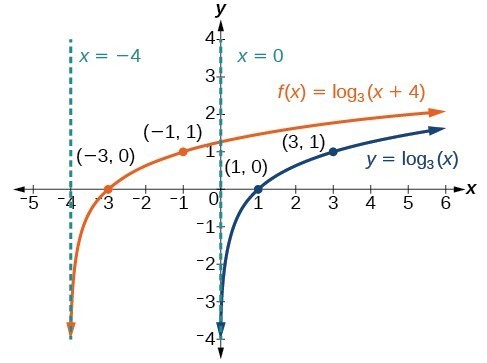Graph of two functions. The parent function is y=log_3(x), with an asymptote at x=0 and labeled points at (1, 0), and (3, 1).The translation function f(x)=log_3(x+4) has an asymptote at x=-4 and labeled points at (-3, 0) and (-1, 1). 5. The domain is [latex]\left(0,\infty \right)[/latex], the range is [latex]\left(-\infty ,\infty \right)[/latex], and the vertical asymptote is x = 0. 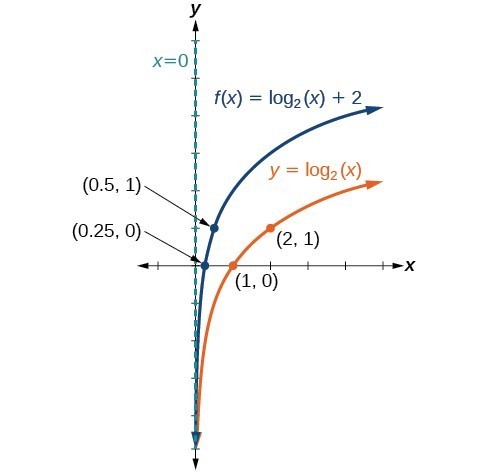Graph of two functions. The parent function is y=log_2(x), with an asymptote at x=0 and labeled points at (1, 0), and (2, 1).The translation function f(x)=log_2(x)+2 has an asymptote at x=0 and labeled points at (0.25, 0) and (0.5, 1). 6. The domain is [latex]\left(0,\infty \right)[/latex], the range is [latex]\left(-\infty ,\infty \right)[/latex], and the vertical asymptote is x = 0. 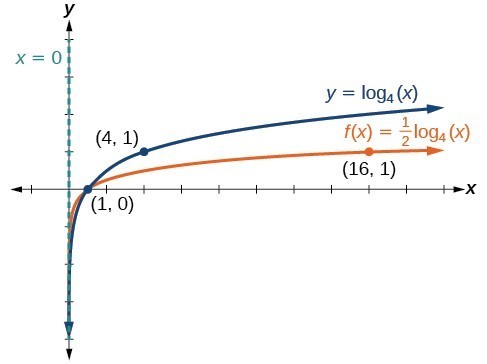Graph of two functions. The parent function is y=log_4(x), with an asymptote at x=0 and labeled points at (1, 0), and (4, 1).The translation function f(x)=(1/2)log_4(x) has an asymptote at x=0 and labeled points at (1, 0) and (16, 1). 7. The domain is [latex]\left(2,\infty \right)[/latex], the range is [latex]\left(-\infty ,\infty \right)[/latex], and the vertical asymptote is x = 2.
Graph of f(x)=3log(x-2)+1 with an asymptote at x=2.
8. The domain is [latex]\left(-\infty ,0\right)[/latex], the range is [latex]\left(-\infty ,\infty \right)[/latex], and the vertical asymptote is x = 0. Graph of f(x)=-log(-x) with an asymptote at x=0.

9. [latex]x\approx 3.049[/latex]

10. x = 1 11. [latex]f\left(x\right)=2\mathrm{ln}\left(x+3\right)-1[/latex]

Solutions to Odd-Numbered Exercises

1. Since the functions are inverses, their graphs are mirror images about the line y = x. So for every point [latex]\left(a,b\right)[/latex] on the graph of a logarithmic function, there is a corresponding point [latex]\left(b,a\right)[/latex] on the graph of its inverse exponential function. 3. Shifting the function right or left and reflecting the function about the y-axis will affect its domain. 5. No. A horizontal asymptote would suggest a limit on the range, and the range of any logarithmic function in general form is all real numbers. 7. Domain: [latex]\left(-\infty ,\frac{1}{2}\right)[/latex]; Range: [latex]\left(-\infty ,\infty \right)[/latex] 9. Domain: [latex]\left(-\frac{17}{4},\infty \right)[/latex]; Range: [latex]\left(-\infty ,\infty \right)[/latex] 11. Domain: [latex]\left(5,\infty \right)[/latex]; Vertical asymptote: x = 5 13. Domain: [latex]\left(-\frac{1}{3},\infty \right)[/latex]; Vertical asymptote: [latex]x=-\frac{1}{3}[/latex] 15. Domain: [latex]\left(-3,\infty \right)[/latex]; Vertical asymptote: x = –3 17. Domain: [latex]\left(\frac{3}{7},\infty \right)[/latex]; Vertical asymptote: [latex]x=\frac{3}{7}[/latex] ; End behavior: as [latex]x\to {\left(\frac{3}{7}\right)}^{+},f\left(x\right)\to -\infty [/latex] and as [latex]x\to \infty ,f\left(x\right)\to \infty [/latex] 19. Domain: [latex]\left(-3,\infty \right)[/latex] ; Vertical asymptote: x = –3; End behavior: as [latex]x\to -{3}^{+}[/latex] , [latex]f\left(x\right)\to -\infty [/latex] and as [latex]x\to \infty [/latex] , [latex]f\left(x\right)\to \infty [/latex] 21. Domain: [latex]\left(1,\infty \right)[/latex]; Range: [latex]\left(-\infty ,\infty \right)[/latex]; Vertical asymptote: x = 1; x-intercept: [latex]\left(\frac{5}{4},0\right)[/latex]; y-intercept: DNE 23. Domain: [latex]\left(-\infty ,0\right)[/latex]; Range: [latex]\left(-\infty ,\infty \right)[/latex]; Vertical asymptote: x = 0; x-intercept: [latex]\left(-{e}^{2},0\right)[/latex]; y-intercept: DNE 25. Domain: [latex]\left(0,\infty \right)[/latex]; Range: [latex]\left(-\infty ,\infty \right)[/latex]; Vertical asymptote: x = 0; x-intercept: [latex]\left({e}^{3},0\right)[/latex]; y-intercept: DNE 27. B 29. C 31. B 33. C 35.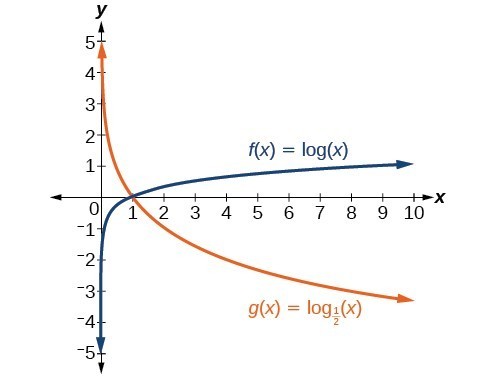 Graph of two functions, g(x) = log_(1/2)(x) in orange and f(x)=log(x) in blue. 37. 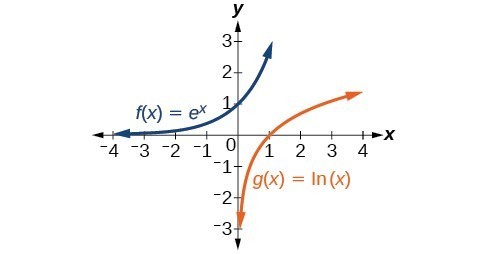Graph of two functions, g(x) = ln(1/2)(x) in orange and f(x)=e^(x) in blue. 39. C 41. 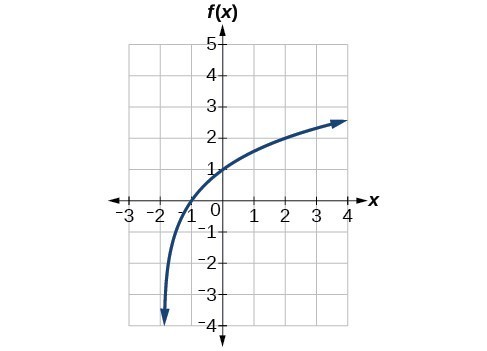Graph of f(x)=log_2(x+2). 43. 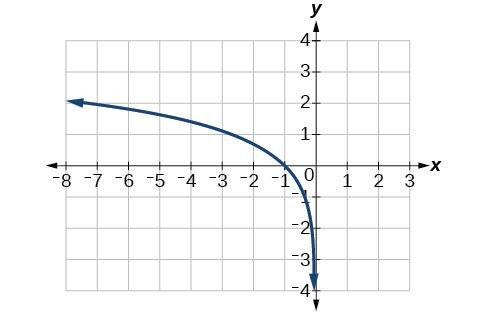Graph of f(x)=ln(-x). 45. 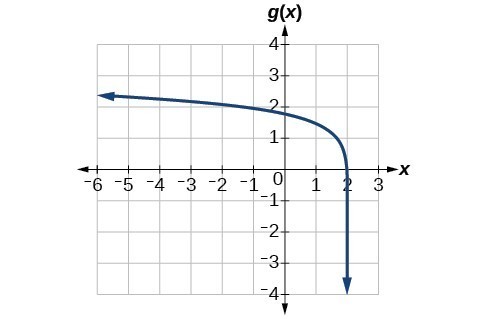Graph of g(x)=log(6-3x)+1. 47. [latex]f\left(x\right)={\mathrm{log}}_{2}\left(-\left(x - 1\right)\right)[/latex] 49. [latex]f\left(x\right)=3{\mathrm{log}}_{4}\left(x+2\right)[/latex] 51. x = 2 53. [latex]x\approx \text{2}\text{.303}[/latex] 55. [latex]x\approx -0.472[/latex] 57. The graphs of [latex]f\left(x\right)={\mathrm{log}}_{\frac{1}{2}}\left(x\right)[/latex] and [latex]g\left(x\right)=-{\mathrm{log}}_{2}\left(x\right)[/latex] appear to be the same; Conjecture: for any positive base [latex]b\ne 1[/latex], [latex]{\mathrm{log}}_{b}\left(x\right)=-{\mathrm{log}}_{\frac{1}{b}}\left(x\right)[/latex]. 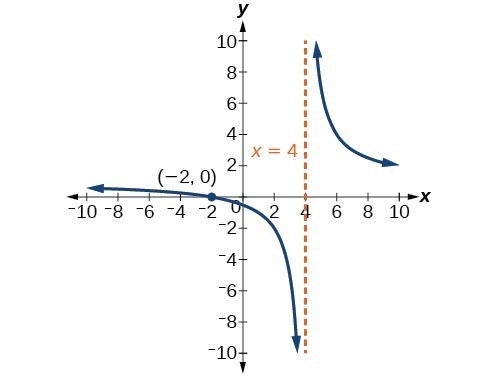59. Recall that the argument of a logarithmic function must be positive, so we determine where [latex]\frac{x+2}{x - 4}>0[/latex] . From the graph of the function [latex]f\left(x\right)=\frac{x+2}{x - 4}[/latex], note that the graph lies above the x-axis on the interval [latex]\left(-\infty ,-2\right)[/latex] and again to the right of the vertical asymptote, that is [latex]\left(4,\infty \right)[/latex]. Therefore, the domain is [latex]\left(-\infty ,-2\right)\cup \left(4,\infty \right)[/latex].

Licenses & Attributions

CC licensed content, Shared previously

  • Precalculus. Provided by: OpenStax Authored by: Jay Abramson, et al.. Located at: https://openstax.org/books/precalculus/pages/1-introduction-to-functions. License: CC BY: Attribution. License terms: Download For Free at : http://cnx.org/contents/[email protected]..