We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > Prealgebra

Identifying Characteristics of Polynomials

Learning Outcomes

  • Identify whether a polynomial is a monomial, binomial, or trinomial
  • Determine the degree of a polynomial
 

Identify Polynomials, Monomials, Binomials, and Trinomials

In Evaluate, Simplify, and Translate Expressions, you learned that a term is a constant or the product of a constant and one or more variables. When it is of the form [latex]a{x}^{m}[/latex], where [latex]a[/latex] is a constant and [latex]m[/latex] is a whole number, it is called a monomial. A monomial, or a sum and/or difference of monomials, is called a polynomial.  

Polynomials

polynomial—A monomial, or two or more monomials, combined by addition or subtraction monomial—A polynomial with exactly one term binomial— A polynomial with exactly two terms trinomial—A polynomial with exactly three terms
  Notice the roots:
  • poly- means many
  • mono- means one
  • bi- means two
  • tri- means three
Here are some examples of polynomials:
Polynomial [latex]b+1[/latex] [latex]4{y}^{2}-7y+2[/latex] [latex]5{x}^{5}-4{x}^{4}+{x}^{3}+8{x}^{2}-9x+1[/latex]
Monomial [latex]5[/latex] [latex]4{b}^{2}[/latex] [latex]-9{x}^{3}[/latex]
Binomial [latex]3a - 7[/latex] [latex]{y}^{2}-9[/latex] [latex]17{x}^{3}+14{x}^{2}[/latex]
Trinomial [latex]{x}^{2}-5x+6[/latex] [latex]4{y}^{2}-7y+2[/latex] [latex]5{a}^{4}-3{a}^{3}+a[/latex]
Notice that every monomial, binomial, and trinomial is also a polynomial. They are special members of the family of polynomials and so they have special names. We use the words ‘monomial’, ‘binomial’, and ‘trinomial’ when referring to these special polynomials and just call all the rest ‘polynomials’.  

example

Determine whether each polynomial is a monomial, binomial, trinomial, or other polynomial: 1. [latex]8{x}^{2}-7x - 9[/latex] 2. [latex]-5{a}^{4}[/latex] 3. [latex]{x}^{4}-7{x}^{3}-6{x}^{2}+5x+2[/latex] 4. [latex]11 - 4{y}^{3}[/latex] 5. [latex]n[/latex] Solution
Polynomial Number of terms Type
1. [latex]8{x}^{2}-7x - 9[/latex] [latex]3[/latex] Trinomial
2. [latex]-5{a}^{4}[/latex] [latex]1[/latex] Monomial
3. [latex]{x}^{4}-7{x}^{3}-6{x}^{2}+5x+2[/latex] [latex]5[/latex] Polynomial
4. [latex]11 - 4{y}^{3}[/latex] [latex]2[/latex] Binomial
5. [latex]n[/latex] [latex]1[/latex] Monomial
 

try it

[ohm_question]146073[/ohm_question]
 

Determine the Degree of Polynomials

In this section, we will work with polynomials that have only one variable in each term. The degree of a polynomial and the degree of its terms are determined by the exponents of the variable. A monomial that has no variable, just a constant, is a special case. The degree of a constant is [latex]0[/latex] —it has no variable.  

Degree of a Polynomial

The degree of a term is the exponent of its variable. The degree of a constant is [latex]0[/latex]. The degree of a polynomial is the highest degree of all its terms.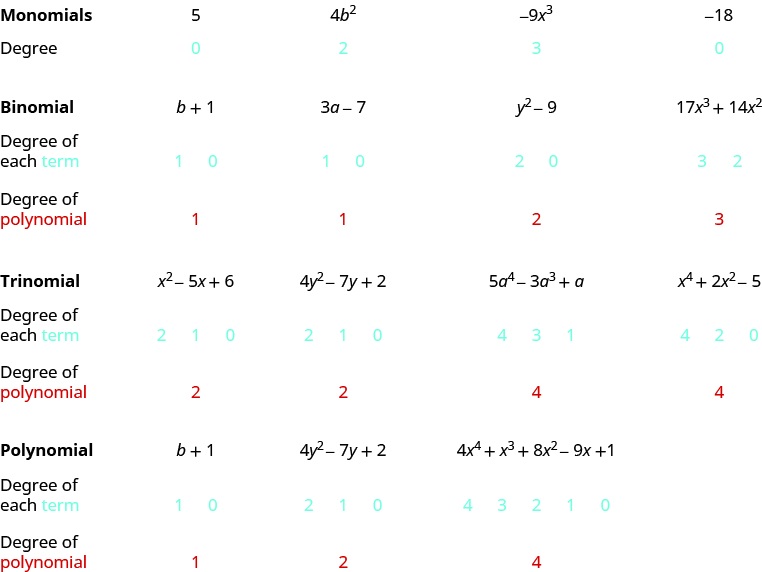
  Let's see how this works by looking at several polynomials. We'll take it step by step, starting with monomials, and then progressing to polynomials with more terms. Remember: Any base written without an exponent has an implied exponent of [latex]1[/latex]. A table is shown. The top row is titled  

example

Find the degree of the following polynomials: 1. [latex]4x[/latex] 2. [latex]3{x}^{3}-5x+7[/latex] 3. [latex]-11[/latex] 4. [latex]-6{x}^{2}+9x - 3[/latex] 5. [latex]8x+2[/latex]

Answer: Solution

1. [latex]4x[/latex]
The exponent of [latex]x[/latex] is one. [latex]x={x}^{1}[/latex] The degree is [latex]1[/latex].
2. [latex]3{x}^{3}-5x+7[/latex]
The highest degree of all the terms is [latex]3[/latex]. The degree is [latex]3[/latex]
3. [latex]-11[/latex]
The degree of a constant is [latex]0[/latex]. The degree is [latex]0[/latex].
4. [latex]-6{x}^{2}+9x - 3[/latex]
The highest degree of all the terms is [latex]2[/latex]. The degree is [latex]2[/latex].
5. [latex]8x+2[/latex]
The highest degree of all the terms is [latex]1[/latex]. The degree is [latex]1[/latex].

 

try it

[ohm_question]146070[/ohm_question]
  Working with polynomials is easier when you list the terms in descending order of degrees. When a polynomial is written this way, it is said to be in standard form. Look back at the polynomials in the previous example. Notice that they are all written in standard form. Get in the habit of writing the term with the highest degree first.  

Licenses & Attributions

CC licensed content, Original

CC licensed content, Specific attribution