We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > ALGEBRA / TRIG I

Graphing Lines Using X- and Y- Intercepts

Learning Outcomes

  • Graph a linear equation using x- and y-intercepts

Graph a Line Using the Intercepts

To graph a linear equation by plotting points, you can use the intercepts as two of your three points. Find the two intercepts, and then a third point to ensure accuracy, and draw the line. This method is often the quickest way to graph a line.

Graph a line using the intercepts

  1. Find the [latex]x-[/latex] and [latex]\text{y-intercepts}[/latex] of the line.
    • Let [latex]y=0[/latex] and solve for [latex]x[/latex]
    • Let [latex]x=0[/latex] and solve for [latex]y[/latex].
  2. Find a third solution to the equation.
  3. Plot the three points and then check that they line up.
  4. Draw the line.
 

example

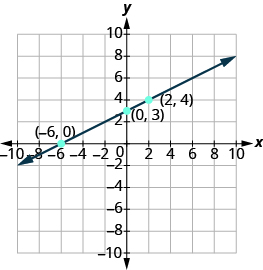
Graph [latex]-x+2y=6[/latex] using intercepts. Solution First, find the [latex]x\text{-intercept}[/latex].

Let [latex]y=0[/latex], [latex-display]\begin{array}{}\\ -x+2y=6\\ -x+2\left(0\right)=6\\ -x=6\\ x=-6\end{array}[/latex-display] The [latex]x\text{-intercept}[/latex] is [latex]\left(-6,0\right)[/latex].

Now find the [latex]y\text{-intercept}[/latex].

Let [latex]x=0[/latex]. [latex-display]\begin{array}{}\\ -x+2y=6\\ -0+2y=6\\ \\ \\ 2y=6\\ y=3\end{array}[/latex-display] The [latex]y\text{-intercept}[/latex] is [latex]\left(0,3\right)[/latex].

Find a third point.

We’ll use [latex]x=2[/latex], [latex-display]\begin{array}{}\\ -x+2y=6\\ -2+2y=6\\ \\ \\ 2y=8\\ y=4\end{array}[/latex-display] A third solution to the equation is [latex]\left(2,4\right)[/latex].

Summarize the three points in a table and then plot them on a graph.
[latex]-x+2y=6[/latex]
x y (x,y)
[latex]-6[/latex] [latex]0[/latex] [latex]\left(-6,0\right)[/latex]
[latex]0[/latex] [latex]3[/latex] [latex]\left(0,3\right)[/latex]
[latex]2[/latex] [latex]4[/latex] [latex]\left(2,4\right)[/latex]
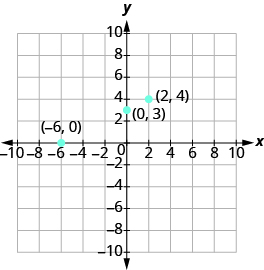The graph shows the x y-coordinate plane. The x and y-axis each run from -10 to 10. Three labeled points are shown at Do the points line up? Yes, so draw line through the points. The graph shows the x y-coordinate plane. The x and y-axis each run from -10 to 10. Three labeled points are shown at

Example

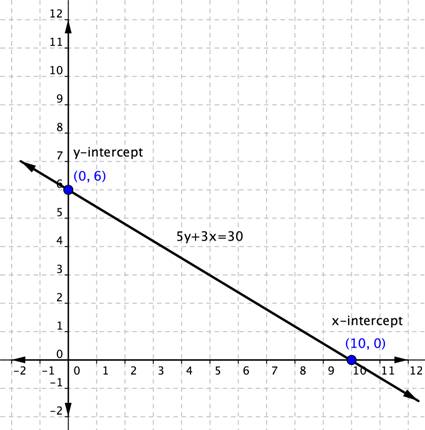
Graph [latex]5y+3x=30[/latex] using the x and y-intercepts.

Answer: When an equation is in [latex]Ax+By=C[/latex] form, you can easily find the x- and y-intercepts and then graph.

[latex]\begin{array}{r}5y+3x=30\\5y+3\left(0\right)=30\\5y+0=30\\5y=30\\y=\,\,\,6\\y\text{-intercept}\,\left(0,6\right)\end{array}[/latex]

To find the y-intercept, set [latex]x=0[/latex] and solve for y.

[latex]\begin{array}{r}5y+3x=30\\5\left(0\right)+3x=30\\0+3x=30\\3x=30\\x=10\\x\text{-intercept}\left(10,0\right)\end{array}[/latex]

To find the x-intercept, set [latex]y=0[/latex] and solve for x.

Answer

 

try it

[ohm_question]146999[/ohm_question]
Watch the following video for more on how to graph a line using the intercepts. https://youtu.be/k8r-q_T6UFk

example

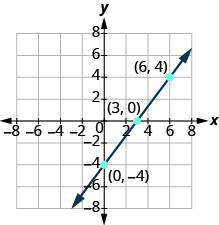
Graph [latex]4x - 3y=12[/latex] using intercepts.

Answer: Solution Find the intercepts and a third point. The figure shows 3 solutions to the equation 4 x - 3 y = 12. The first is titled We list the points and show the graph.

[latex]4x - 3y=12[/latex]
[latex]x[/latex] [latex]y[/latex] [latex]\left(x,y\right)[/latex]
[latex]3[/latex] [latex]0[/latex] [latex]\left(3,0\right)[/latex]
[latex]0[/latex] [latex]-4[/latex] [latex]\left(0,-4\right)[/latex]
[latex]6[/latex] [latex]4[/latex] [latex]\left(6,4\right)[/latex]
The graph shows the x y-coordinate plane. Both axes run from -7 to 7. Three unlabeled points are drawn at

 

try it

[ohm_question]146998[/ohm_question]
In the next example, there is only one intercept because the line passes through the point (0,0).

example

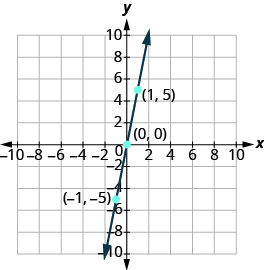
Graph [latex]y=5x[/latex] using the intercepts.

Answer: Solution The figure shows 2 solutions to y = 5 x. The first solution is titled This line has only one intercept! It is the point [latex]\left(0,0\right)[/latex]. To ensure accuracy, we need to plot three points. Since the intercepts are the same point, we need two more points to graph the line. As always, we can choose any values for [latex]x[/latex], so we’ll let [latex]x[/latex] be [latex]1[/latex] and [latex]-1[/latex]. The figure shows two substitutions in the equation y = 5 x. In the first substitution, the first line is y = 5 x. The second line is y = 5 open parentheses 1, shown in red, closed parentheses. The third line is y =5. The last line is Organize the points in a table.

[latex]y=5x[/latex]
[latex]x[/latex] [latex]y[/latex] [latex]\left(x,y\right)[/latex]
[latex]0[/latex] [latex]0[/latex] [latex]\left(0,0\right)[/latex]
[latex]1[/latex] [latex]5[/latex] [latex]\left(1,5\right)[/latex]
[latex]-1[/latex] [latex]-5[/latex] [latex]\left(-1,-5\right)[/latex]
Plot the three points, check that they line up, and draw the line. The graph shows the x y-coordinate plane. The x and y-axis each run from -10 to 10. A line passes through three labeled points,

 

try it

[ohm_question]147001[/ohm_question]
In the following video we show another example of how to plot a line using the intercepts of the line. https://youtu.be/Wgpq0zO6z3o

Choosing the Most Convenient Way to Graph a Line Given an Equation

While we could graph any linear equation by plotting points, it may not always be the most convenient method. This table shows six of equations we’ve graphed in this chapter, and the methods we used to graph them.
Equation Method
#1 [latex]y=2x+1[/latex] Plotting points
#2 [latex]y=\Large\frac{1}{2}\normalsize x+3[/latex] Plotting points
#3 [latex]x=-7[/latex] Vertical line
#4 [latex]y=4[/latex] Horizontal line
#5 [latex]2x+y=6[/latex] Intercepts
#6 [latex]4x - 3y=12[/latex] Intercepts
What is it about the form of equation that can help us choose the most convenient method to graph its line? Notice that in equations #1 and #2, y is isolated on one side of the equation, and its coefficient is 1. We found points by substituting values for x on the right side of the equation and then simplifying to get the corresponding y- values. Equations #3 and #4 each have just one variable. Remember, in this kind of equation the value of that one variable is constant; it does not depend on the value of the other variable. Equations of this form have graphs that are vertical or horizontal lines. In equations #5 and #6, both x and y are on the same side of the equation. These two equations are of the form [latex]Ax+By=C[/latex] . We substituted [latex]y=0[/latex] and [latex]x=0[/latex] to find the x- and y- intercepts, and then found a third point by choosing a value for x or y. This leads to the following strategy for choosing the most convenient method to graph a line.

Choose the most convenient method to graph a line

  1. If the equation has only one variable. It is a vertical or horizontal line.
    • [latex]x=a[/latex] is a vertical line passing through the [latex]x\text{-axis}[/latex] at [latex]a[/latex]
    • [latex]y=b[/latex] is a horizontal line passing through the [latex]y\text{-axis}[/latex] at [latex]b[/latex].
  2. If [latex]y[/latex] is isolated on one side of the equation. Graph by plotting points.
    • Choose any three values for [latex]x[/latex] and then solve for the corresponding [latex]y\text{-}[/latex] values.
  3. If the equation is of the form [latex]Ax+By=C[/latex], find the intercepts.
    • Find the [latex]x\text{-}[/latex] and [latex]y\text{-}[/latex] intercepts and then a third point.
 

example

Identify the most convenient method to graph each line: 1. [latex]y=-3[/latex] 2. [latex]4x - 6y=12[/latex] 3. [latex]x=2[/latex] 4. [latex]y=\frac{2}{5}x - 1[/latex] Solution 1. [latex]y=-3[/latex] This equation has only one variable, [latex]y[/latex]. Its graph is a horizontal line crossing the [latex]y\text{-axis}[/latex] at [latex]-3[/latex]. 2. [latex]4x - 6y=12[/latex] This equation is of the form [latex]Ax+By=C[/latex]. Find the intercepts and one more point. 3. [latex]x=2[/latex] There is only one variable, [latex]x[/latex]. The graph is a vertical line crossing the [latex]x\text{-axis}[/latex] at [latex]2[/latex]. 4. [latex]y=\Large\frac{2}{5}\normalsize x - 1[/latex] Since [latex]y[/latex] is isolated on the left side of the equation, it will be easiest to graph this line by plotting three points.
 

try it

[ohm_question]147002[/ohm_question]

Contribute!

Did you have an idea for improving this content? We’d love your input.

Licenses & Attributions

CC licensed content, Original

  • Question ID 147001, 146998, 146999, 147002. Authored by: Lumen Learning. License: CC BY: Attribution.

CC licensed content, Specific attribution